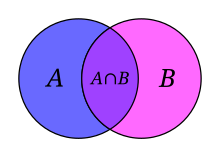
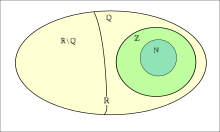
Lập luận đường chéo của Cantor
|
Read other articles:

FST Наявні структури PDBПошук ортологів: PDBe RCSB Список кодів PDB 3HH2, 2B0U, 2P6A Ідентифікатори Символи FST, FS, follistatin Зовнішні ІД OMIM: 136470 MGI: 95586 HomoloGene: 7324 GeneCards: FST Онтологія гена Молекулярна функція • heparan sulfate proteoglycan binding• GO:0001948, GO:0016582 protein binding• signal transducer activity• activin binding• activin receptor antagonist ...

Golf tournament Magical Kenya OpenTournament informationLocationNairobi, KenyaEstablished1967Course(s)Muthaiga Golf ClubPar71Length7,228 yards (6,609 m)Tour(s)European TourChallenge TourSafari CircuitFormatStroke playPrize fundUS$2,000,000Month playedMarchTournament record scoreAggregate263 Justin Harding (2021)To par−21 as aboveCurrent champion Jorge CampilloLocation MapMuthaiga GCLocation in Kenya The Kenya Open, currently titled as the Magical Kenya Open for sponsorship reasons, is ...

S-Bahn Berlin البلد ألمانيا محطات القطارات 166[1] الموقع الرسمي الموقع الرسمي تعديل مصدري - تعديل برلين إس باهن هو نظام سكة حديد سريع للنقل في برلين وحولها، عاصمة ألمانيا. تم تشغيله تحت هذا الاسم منذ ديسمبر 1930، بعد أن تم تسميته سابقًا بمنطقة التعريفة الخاصة Berliner Sta...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (فبراير 2017) إيرين ثورن معلومات شخصية الميلاد 19 مايو 1981 (العمر 42 سنة)أوريم الطول 178 سنتيمتر مركز اللعب مدافع مسدد الهدف الجنسية الولايات المتحدة الوزن 68 كيلو

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (ديسمبر 2018) لوري آدامز معلومات شخصية الميلاد 14 فبراير 1931 (92 سنة) مركز اللعب جناح [لغات أخرى] الجنسية المملكة المتحدة المسيرة الاحترافية1 سنوات فريق م. (

Voce principale: Storia della Sicilia. Questa voce è parte della serieStoria della Sicilia Preistoria Preistoria della Sicilia Sicilia preellenica Storia antica Sicilia fenicia Sicilia greca Sicilia punica Sicilia romana Storia medievale Sicilia vandala Sicilia erulo-ostrogota Sicilia bizantina Conquista islamica della Sicilia Sicilia islamica Conquista normanna dell'Italia meridionale Contea di Sicilia Sicilia normanna Sicilia sveva Sicilia angioina Sicilia aragonese Storia moderna Sicilia ...

Former currency of the Kingdom of Westphalia This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Westphalian frank – news · newspapers · books · scholar · JSTOR (May 2008) (Learn how and when to remove this template message) A gold 10 Franken coin The Westphalian frank was a currency of the Kingdom of Westphalia...

July 2022 missile attack in Ukraine Serhiivka missile strikePart of the 2022 Russian invasion of UkraineThe residential building after the strikeLocationSerhiivka, Serhiivka settlement hromada, Bilhorod-Dnistrovskyi Raion, Odesa Oblast, UkraineDate1 July 2022 01:00 AM[1] (UTC+3)TargetResidential building and recreation facilityAttack typeMissile strikeDeaths21 (including 1 child)[2]Injured38 (including 6 children)[2]Perpetrators Russian Air Force vteRussian invasi...

Radio station in Bennington, NebraskaKFFFBennington, NebraskaBroadcast areaOmaha-Council BluffsFrequency93.3 MHz (HD Radio)Branding93.3 The WolfProgrammingFormatFM/HD1: Classic countrySubchannelsHD2: Regional Mexican 102.3 El PatronOwnershipOwneriHeartMedia(iHM Licenses, LLC)Sister stationsKFAB, KGOR, KISO, KXKTHistoryFirst air date1991; 32 years ago (1991) (as KRRK)Former call signsKRRK (1989–1996)KTNP (1996–2000)KRQC (2000–2004)KHUS (2004–2008)KTWI (2008–2012)Tec...

Japanese manga series For other uses, see Bananafish (disambiguation). Banana FishFirst tankōbon volume cover,featuring Ash LynxGenreDrama, thriller[1]Created byAkimi Yoshida MangaWritten byAkimi YoshidaPublished byShogakukanEnglish publisherNA: Viz MediaImprintFlower ComicsMagazineBessatsu Shōjo ComicEnglish magazineNA: Pulp, Animerica ExtraDemographicShōjoOriginal runMay 1985 (1985-05) – April 1994 (1994-04)Volumes19 (List of volumes) Prequels...

American physicist (1908–1991) Milton Spinoza PlessetCopenhagen 1963Born(1908-02-07)February 7, 1908Pittsburgh, PennsylvaniaDiedFebruary 19, 1991(1991-02-19) (aged 83)CitizenshipUnited StatesAlma materUniversity of Pittsburgh Yale UniversityKnown forRayleigh-Plesset equation Møller–Plesset perturbation theoryAwardsASME Fluids Engineering Award[1]Scientific careerFieldsFluid dynamicsInstitutionsUniversity of Rochester California Institute of TechnologyDoctoral advis...

西班牙廣場,右前方的黃白色建築曾經是詩人約翰·濟慈的住所 西班牙廣場(義大利語:Piazza di Spagna)是位于義大利羅馬的廣場,旁有羅馬地鐵的同名車站。山上天主圣三堂(由法國波旁王朝的國王所資助建造)就位在與西班牙廣場相接的西班牙階梯頂端。西班牙廣場也曾經出現在電影《羅馬假期》的場景中。 設計 西班牙廣場上有一個巴洛克藝術的噴泉,被稱為破船噴泉�...

This article uses bare URLs, which are uninformative and vulnerable to link rot. Please consider converting them to full citations to ensure the article remains verifiable and maintains a consistent citation style. Several templates and tools are available to assist in formatting, such as reFill (documentation) and Citation bot (documentation). (August 2022) (Learn how and when to remove this template message) Village in Southwestern, North MacedoniaRadožda РадождаVillageRadoždaLocat...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Egypt–Russia relations – news · newspapers · books · scholar · JSTOR (February 2014) (Learn how and when to remove this template message) Bilateral relationsEgypt–Russia relations Egypt Russia Diplomatic missionEgyptian Embassy, MoscowRussian Embassy, Cairo...

Novel by Anchee Min This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: Becoming Madame Mao – news · newspapers · books · scholar · JSTOR (February 2011) (Learn how and when to remove this template message...

2009 single by the Pussycat Dolls (either solo or featuring Snoop Dogg or Devolo)Bottle PopSingle by the Pussycat Dolls (either solo or featuring Snoop Dogg or Devolo)from the album Doll Domination ReleasedFebruary 23, 2009 (2009-02-23)Studio Chalice Recording (Los Angeles, California) Record Plant (Hollywood, California) Irvine Spot (Irvine, California) GenreElectronicaLength3:30LabelInterscopeSongwriter(s) Sean The Pen Garrett Fernando Garibay Nicole Scherzinger Producer(s) G...

American radio broadcasting company Alpha Media LLCTrade nameAlpha MediaIndustryMass mediaGenreRadio broadcastingPredecessorAlpha BroadcastingL&L Broadcasting LLCMain Line BroadcastingTriad BroadcastingFoundedPortland, Oregon (2014 (2014))FounderLawrence R. Larry WilsonHeadquartersPortland, Oregon, USNumber of locations135[1]Key peopleLarry Wilson (Chairman)Bob Proffitt (President/CEO)Donna Heffner (CFO)Websitewww.alphamediausa.com Alpha Media LLC is a radio broadcasting comp...

American comedy television series The ComediansGenre Comedy Mockumentary Based onUlveson och Herngren by Felix Herngren & John NordlingDeveloped by Larry Charles Billy Crystal Matt Nix Ben Wexler Starring Billy Crystal Josh Gad Stephnie Weir Matt Oberg Dana Delany Megan Ferguson Country of originUnited StatesOriginal languageEnglishNo. of seasons1No. of episodes13 (list of episodes)ProductionExecutive producers Larry Charles Jennifer Corey Billy Crystal Matt Nix Ben Wexler Henrik Bastin M...

中華民國 中華民國政府与政治系列条目 政府(沿革) 憲法 中華民國憲法(憲政史) 中華民國憲法增修條文 總統及副總統在臺灣之直接選舉與罷免 國會在臺灣之全面選舉與罷免 國民大會(已凍結) 一府五院 總統 總統:蔡英文 副總統:賴清德 總統府 秘書長:林佳龍 資政 國策顧問 戰略顧問 國史館 中央研究院 國家安全會議 主席:蔡英文 副主席:賴清德 秘書長:顧立雄...

Indian film actress (b. 1990) Ishita Raj SharmaSharma in 2022Born (1990-07-12) 12 July 1990 (age 33)Mumbai, India[1]OccupationsActressModelYears active2011–presentKnown forPyaar Ka Punchnama Pyaar Ka Punchnama 2 Ishita Raj Sharma (born 12 July 1990) is an Indian actress and model,[2] best known for her work in Pyaar Ka Punchnama, Pyaar Ka Punchnama 2 and Sonu Ke Titu Ki Sweety.[3][4] Education Ishita studied at Delhi Public School, Mathura Road...